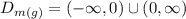Question

Composition of functions, interval notation

Answer 1

Given the functions:

`\begin{gathered} g(x)=\frac{1}{\sqrt[]{x}} \\ m(x)=x^2-4 \end{gathered}`

I would like to find their domain as well and then complete the answers:

`\begin{gathered} D_g=(0,\infty) \\ D_m=(-\infty,\infty) \end{gathered}`

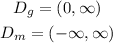
For the first question: g(x) / m(x)

`\begin{gathered} (g(x))/(m(x))=\frac{\frac{1}{\sqrt[]{x}}}{x^2-4}=\frac{1}{\sqrt[]{x}\cdot(x^2-4)}=\frac{1}{x-4\sqrt[]{x}} \\ x-4\sqrt[]{x}\\e0 \\ x\\e4\sqrt[]{x} \\ x^2\\e4x \\ x\\e4 \end{gathered}`

As we can see, the domain of this function cannot take negative values nor 4, 0. So, its domain is

`D_{(g)/(m)}=(0,4)\cup(4,\infty)`

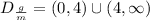
For the second domain g(m(x)), let's find out what is the function:

`\begin{gathered} g(m(x))=\frac{1}{\sqrt[]{x^2-4}} \\ \sqrt[]{x^2-4}>0 \\ x^2>4 \\ x>2 \\ x<-2 \end{gathered}`

This means that x cannot be among the interval -2,2:

`D_(g(m))=(-\infty,-2)\cup(2,\infty)`

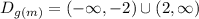
For the last domain m(g(x)) we perfome the same procedure:

`m(g(x))=(\frac{1}{\sqrt[]{x}})^2-4=(1)/(x)-4`

For this domain it is obvious that x cannot take the zero value but anyone else.

`D_(m(g))=(-\infty,0)\cup(0,\infty)_{}`