Question

If A/B and C/D are rational expressions,then which of the following is true?*PHOTO*

Answer 1

In general,

`\begin{gathered} (w)/(x)*(y)/(z)=(w*y)/(x*z) \\ x,z\\e0 \end{gathered}`

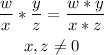
Therefore, in our case, (Notice that since A/B and C/D are rational expressions, B and D cannot be equal to zero)

`(A)/(B)*(C)/(D)=(A*C)/(B*D)`

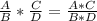
Notice that the left side of each option includes the term

`(A)/(B)*(D)/(C)`

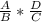
However, we cannot assure that C is different than zero because it is only stated that C/D is rational.

Furthermore,

`(A)/(B)*(D)/(C)=(A*D)/(B*C)`

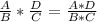
And (A*D)/(B*C) is not included among the options.

Therefore, the answer has to be option D as it is the only one that correctly expresses the multiplication of two fractions.

Remember that there is a mistake in each option, the left side has to be A/B*D/C