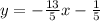The equation for the line in point-slope form is:

Where m is the slope and (x1, y1) is a point of the line. If we have two points (x1,y1) and (x2, y2), the slope is equal to:

So, replacing (3, -8) and (-2, 5), we get that the slope and the equation of the line are:
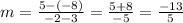
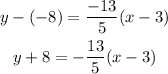
Therefore, the equation in slope-intercept form is calculated as:
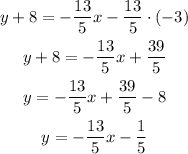
Answer: Point-slope form:

slope-intercept form: