Question

The vertex of the parabola below is at the point

Answer 1

The equation of a parabola in a vertex form is given

since the parabola is on the x-axis.

`\begin{gathered} x=a(y-h)^2+k \\ \text{Where } \\ \text{Vertex}=(h,k) \end{gathered}`

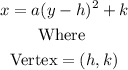
From the diagram given, we have

`\text{vertex}=(-4,-2)`

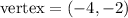
Substituting into the formula above, we have

`\begin{gathered} x=a(y-h)^2+k \\ h=-4,k=-2 \end{gathered}`

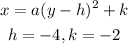
We have

`\begin{gathered} x=(y-(-2)^2-4 \\ x=(y+2)^2-4 \end{gathered}`

Since the parabola is a reflection from the parent function, then

`a=-2`

The equation of the parabola becomes

`x=-2(y+2)^2-4`

Answer; x = -2(y + 2)^2-4