We want to know which ordered pair is a solution of the system of inequalities shown:
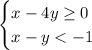
For doing so, we will try to solve both inequalities for one variable, in this case, we will use y.
On the first equation:
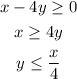
On the second equation:
![\begin{gathered} x-y<-1 \\ x+1-y<0 \\ x+1And joining those two results we get:[tex]x+1Now we check each of the ordered pairs, if they hold the condition above:<p><strong>For (0, 2)</strong></p><p>We have that x=0, and y=2. Thus, </p>[tex]\begin{gathered} x+1=1 \\ (x)/(4)=0 \\ \text{And as }2>0,\text{ (0, 2) is NOT a solution of the system.} \end{gathered}]()
For (-3, 8)
In this case, x=-3 and y=8.
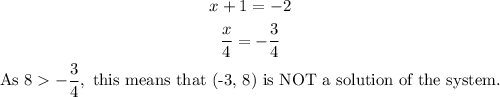
For (2,5)
In this case, x=2 and y=5.
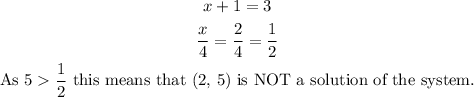
For (-7, -4)
In this case, x=-7 and y=-4.
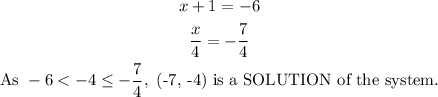
For (6, -1)
We have that x=6 and y=-1.
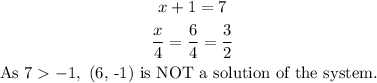
Thus, the ordered pair which is a solution of the system is (-7, -4).