1. slope= 2
2. slope=2
3. slope= 1/3
4. slope indefinite, vertical line
Step-by-step explanation
Step 1
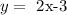
the equation is given in slope(m) - intercept(b)
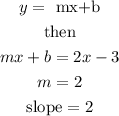
Step 2
when you have two points of a line, P1 and P2 the slope is given by:
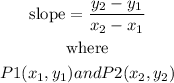
Let
P1(-2,-4) P2(-1,-2)
replace
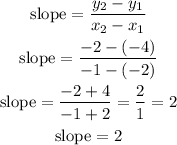
Step 3
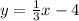
similar to the #1. ,the equation is given in slope(m) - intercept(b)
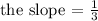
Step 4
let
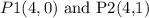
![\begin{gathered} \text{slope}=(y_2-y_1)/(x_2-x_1) \\ \text{slope}=(1-0)/(4-4)=(1)/(0)=\text{indefined} \\ it\text{ means the line is vertical} \end{gathered}]()