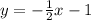Question

Write the linear equation that passes through the two given points (2,-2) and (0,-1)

Answer 1

Given the points:

(x1, y1) ==> (2, -2)

(x2, y2) ==> (0, -1)

To find the linear equation, use the form:

y = mx + b

where m is the slope.

To find the slope, use the formula below:

`m=(y2-y1)/(x2-x1)`

Thus, we have the slope as:

`m=(-1-(-2))/(0-2)=(-1+2)/(0-2)=(1)/(-2)=-(1)/(2)`

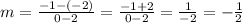
Input 2 for x, -2 for y, and -1/2 for b to find b.

`\begin{gathered} -2=-(1)/(2)(2)+b \\ \\ -2=-1+b \\ \\ -2+1=b \\ \\ -1=b \end{gathered}`

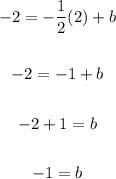
Therefore, the linear equation is:

`y=-(1)/(2)x-1`

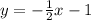
ANSWER:

`y=-(1)/(2)x-1`