ANSWER
It will take 18 months before the company starts making a profit.
Explanation
Given information
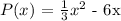
Where x is the number of months.
Step 1: Make P(x) = 0
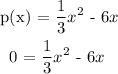
Step 2: Find x from the above equation

Therefore, it will take 18 months before the company starts making a profit.