Given: The following
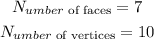
To Determine: The number of edges
Solution:
The Euler's formula is given as
F + V = E + 2,
where F is the number of faces,
V the number of vertices, and
E the number of edges.
Substitute the given into the formula
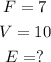
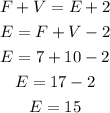
Hence, the number of edges possessed by the polyhedron is 15