Solution:
Remember, distance traveled is the rate times the time. (d = rt) Mrs. Cunningham will overtake Mr. Cunningham when they have traveled the same distance.
Mrs. Cunningham's equation will be:

Since he was traveling 3 hours longer, Mr. Cunningham's equation will be:
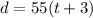
If they travel the same distance, the equations can be set equal to each other:

applying the distributive property, this is equivalent to:
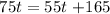
this is equivalent to:
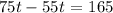
this is equivalent to:

solving for t, we obtain:

So that, we can conclude that the correct answer is:
It will take Mrs. Cunningham 8.25 hours to overtake her husband.