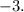To identify the slope and y-intercept, we will take the given equation to its slope-intercept form:
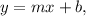
where m is the slope and b is the y-intercept.
To take the equation to its slope-intercept form, we add 3y to the given equation:
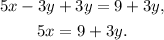
Now, we subtract 9, and get:
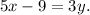
Finally, dividing by 3, we get:
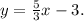
Therefore, the slope and y-intercept are:
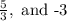
correspondingly.
Answer:
Slope:
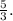
Y-intercept: