Given:
a.) The length of a rectangle is 2 inches more than its width.
Since the length of a rectangle is 2 inches more than its width, we can say that,
Width = W
Length = L = W + 2
Determine the width with respect to its Perimeter, we get:

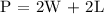
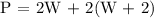
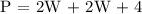
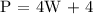
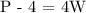
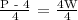

Therefore, the answer is D.