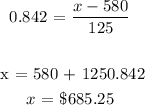Answer:
Step-by-step explanation:
Here, we want to get the amount of money that would guarantee that a person has more money than 80%
That means the probability is greater than 80% or 0.8
Thus, we need to get the z-score that corresponds to this probability
Using a z-score table, we can get this as follows:
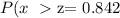
We will now get the value from the obtained z-score
Mathematically:
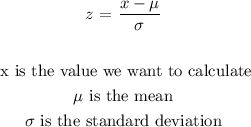
Substituting the values, we have it that: