For this problem, we are given the following rational function:
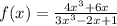
We need to determine the horizontal asymptote for this function. In order to determine this, we need to calculate the limit of the function when x approaches infinity. We have:

The horizontal asymptote exists at y= 4/3. The correct option is C.