Question

Larry Mitchell invested part of his $17000 advance at 2% annual simple interest and the rest at 5% annual simple interest. If his total yearly interest from both accounts was $610, find the amount invested at each rate

Answer 1

The simple interest is given by:

`SI=Prt`

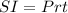
where P is the principal (the amount we invest in the account), r is the interest rate and t is the time of investment.

Let P be the interest Larry made in the 2% account, the simple interest in this case is given by:

`0.02P`

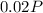
Now for the second account we would have an envestment of (17000-P), then the simple interest have to be:

`0.05(17000-P)`

and we know that both investments have to be equal to 610, then we have:

`\begin{gathered} 0.02P+0.05(17000-P)=610 \\ 0.02P+850-0.05P=610 \\ -0.03P=610-850 \\ -0.03P=-240 \\ P=(-240)/(-0.03) \\ P=8000 \end{gathered}`

Therefore Larry invested $8000 in the 2% account and $9000 in the 5% account.