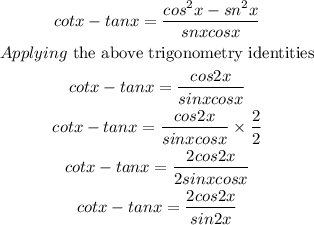
Solution
Box 1 : Apply Quotient Identities
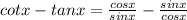
The answer for the first box is

Box 2: Write as one expression
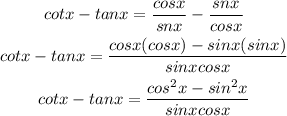
The answer for the second box is
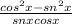
Before the box 3, please note the identity
Note: Trigonometry I dentities
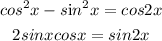
Box 3: Apply Double - Number Identities