We use the formula for the area "A" of a square with equal sides of length "l":
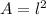
Since we know the value of the area is:
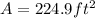
We substitute that value into the formula for the area:
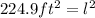
Next, we take the square root of both sides of the equation:
![\sqrt[]{224.9ft^2}=\sqrt[]{l^2}](https://img.qammunity.org/2023/formulas/mathematics/college/omgv9zxe5t79y9e1otzsb9552qlil924dk.png)
On the right side, the exponent and the square root will cancel, and we will get "l", and on the left side, we calculate the square root:

Which can be rounded to the closest value, that is 15 ft.
Answer: 15 feet