Question

Find the sum of the first nine terms of the geometric series 1 – 3 + 9 - 27+....

Answer 1

Hello there. To solve this question, we'll have to remember some properties about geometric series.

Given that we want the sum of

`1-3+9-27...`

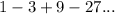
First, we find the general term of this series:

Notice they are all powers of 3, namely

`\begin{gathered} 1=3^0 \\ 3=3^1 \\ 9=3^2 \\ 27=3^3 \\ \vdots \end{gathered}`

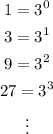
But this is an alternating series, hence the general term is given by:

`a_n=\left(-3\right)^(n-1)`

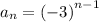
Since we just want the sum of the first 9 terms of this geometric series, we apply the formula:

`S_n=(a_1\cdot\left(1-q^n\right?)/(1-q)`

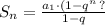
Where q is the ratio between two consecutive terms of the series.

We find q as follows:

`q=(a_2)/(a_1)=(\left(-3\right)^(2-1))/(\left(-3\right)^(1-1))=(-3)/(1)=-3`

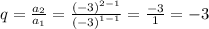
Then we plug n = 9 in the formula, such that:

`S_9=(1\cdot\left(1-\left(-3\right)^9\right?)/(1-\left(-3\right))=(1-\left(-19683\right))/(1+3)=(19684)/(4)`

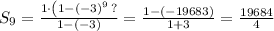
Simplify the fraction by a factor of 4

`S_9=4921`

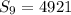
This is the sum of the nine first terms of this geometric series and it is the answer contained in the second option.