Question

How much heat in kcal must be added to 0.68 kg of water at room temperature (20°C) to raise its temperature to 45°C?answer:____ kcal

Answer 1

Given:

Mass, m = 0.68 kg

Initial temperature, T1 = 20°C

Final temperature, T2 = 45°C

Let's find the amount of heat needed.

Apply the Specific Heat Capacity formula:

`\begin{gathered} Q=mc\Delta T \\ \\ Q=mc(T_2-T_1) \end{gathered}`

Where:

m is the mass = 0.68 kg

c is the specific heat of water = 4.187 J/kg °C−1

T1 = 20°C

T2 = 45°C

Plug in the values and solve for Q:

`\begin{gathered} Q=0.68*4.187*(45-20) \\ \\ Q=2.84716(25) \\ \\ Q=71.179\text{ kJ} \end{gathered}`

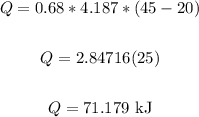
Where:

1 kJ = 0.239 kCal

Since the answer is to be in kCal, we have:

`Q=71.179*0.239=17.01\text{ kCal}`

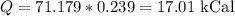
Therefore, the amount if heat added is 17.01 kCal.

ANSWER:

17.01 kCal