To determine the equation of the quadratic equation, which is a parabola, we substitute the coordinates of the vertex of the parabola (h,k) into the general equation.

From the given, notice that the only value of f(x) that does not repeat is 8. This means that the vertex is at (7,8).
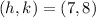
Thus, we only need to obtain the value of a.
Substitute the coordinate of a point (x,y) into the equation and the vertex as well. In this case, let us use the first given point, (5,-4).
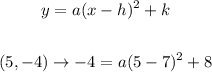
Simplify the obtained equation.
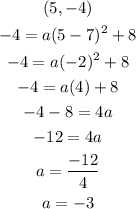
Now that we have the value of a, substitute the coordinates of the obtained vertex and the value of a into the equation of the quadratic equation.
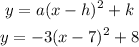
To check, the graph of the given function is as follows:
Therefore, the equation of the quadratic equation is y=-3(x-7)²+8.