Question

The critical angle for a certain liquid-air surface is 20°. What is the index of refraction of this liquid?

Answer 1

`\begin{equation*} 2.92 \end{equation*}`

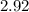
Step-by-step explanation

To find the index of refraction of the liquid, we have to apply the formula for critical angle:

`\theta=\sin^(-1)((n_r)/(n_i))`

where nr = refractive index of air = 1

ni = refractive index of liquid

Hence, by substituting the given values into the equation, we have that the index of refraction of the liquid is:

`\begin{gathered} 20=\sin^(-1)((1)/(n_i)) \\ \sin20=(1)/(n_i) \\ n_i=(1)/(\sin20) \\ n_i=2.92 \end{gathered}`

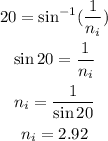
That is the answer.