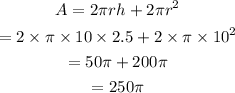ANSWER

Step-by-step explanation
For the first cylinder;
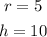
Recall, the formula for calculating the surface area of a cylinder is;
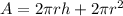
Now, substitute the values for the first cylinder;
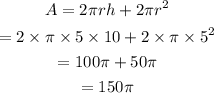
The volume of the first cylinder is calculated using the formula;
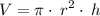
Substitute the values of r and h for the first cylinder;
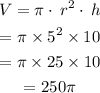
To get the surface area of the second cylinder, we need to calculate the height (h).
To get the height, we use the volume of the first cylinder to get the height of the second (since they have the same volume).
Hence;
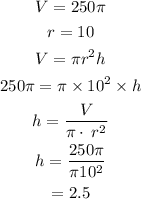
Substitute the height to calculate the surface area is calculated thus;