Question

Find the value of tan A in simplest radical form

Answer 1

In the given right angle triangle BCA : BC = 5, CA = 3 and BA = root 34

From the trignometric ratio of right angle triangle :

The tangent of angle is the ratio of the Adjacent side to the opposite side

`\tan \theta=\frac{Opposite\text{ side}}{Adjacent\text{Side}}`

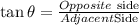
In the given triangle, the side opposite to angle A = BC and adjacent side CB

Substitute the value :

`\begin{gathered} \tan \theta=\frac{Opposite\text{ side}}{Adjacent\text{Side}} \\ \tan A=(BC)/(CB) \\ \tan A=(5)/(3) \\ \tan A=1.66 \\ \\ ^{} \end{gathered}`

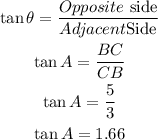
The value of tanA = 5/3 or 1.66