Question

What is the pressure, in mmHg, of a 4.50 g sample of O2 gas, which has a temperature of 33.0 °C, and a volume of 2600 mL?

Answer 1

We are going to assume that the gas behaves like an ideal gas, so we can apply the following equation:

`\begin{gathered} PV=nRT \\ P=(nRT)/(V) \end{gathered}`

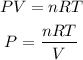
Where,

P is the pressure of the gas in atm

V is the volume of the gas in liters, 2600mL=2.6L

n is the number of moles

R is a constant, 0.08206atm.L/mol.K

T is the temperature in Kelvin, 33.0°C=306.15K

Now we need to find the moles of O2 to plug into the equation. We divide the grams by the molar mass of O2. The molar mass of O2 is 31.9988g/mol.

`\begin{gathered} molO_2=4.50g*(1molO_2)/(MolarMass,gO_2) \\ molO_2=4.50g*(1molO_2)/(31.9988gO_2)=0.140molO_2 \end{gathered}`

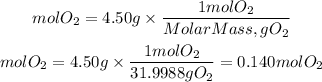
We substitute the values in the ideal gas equation:

`\begin{gathered} P=(nRT)/(V) \\ P=(0.140molO_2*0.08206(atm.L)/(mol.K)*306.15K)/(2.6L) \\ P=1.36atm \end{gathered}`

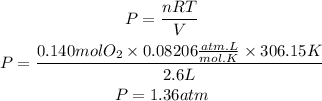
Now, we convert the atm to mmHg:

`\begin{gathered} P=1.36atm*(760mmHg)/(1atm) \\ P=1033mmHg \end{gathered}`

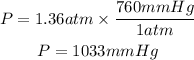
Answer: The pressure of the gas is 1033mmHg