Question

Please I really need help. I just need the answer no steps

Answer 1

Step-by-step explanation

The question wants us to obtain the margin of error

A margin of error tells you how many percentages points your results will differ from the real population value.

The formula to be used is

To do so, we will have to list out the parameters to be used

`\begin{gathered} standard\text{ deviation=}\sigma=13.8 \\ sample\text{ size=n=18} \\ confidence\text{ level=}\gamma=80\text{ \%} \end{gathered}`

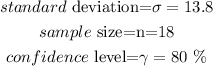
The next step will be to find the z-score value for a confidence level of 80%.

From the statistical table, we have

`Z_(\gamma)=1.28`

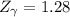
So, we can input the given data obtained into the formula

So we will have

`\begin{gathered} MOE=1.28*\sqrt{(13.8^2)/(18)} \\ \\ MOE=1.28*(13.8)/(√(18)) \\ \\ MOE=1.28*3.2527 \\ \\ MOE=4.16344 \end{gathered}`

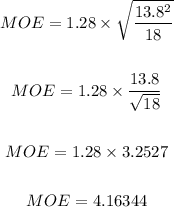
So the margin of error (M.E.) = 4.163 (To 3 decimal places)