Question

Find the equation of the line that is perpendicular to y= -1 over 5x-3 and contains the point (1,2)

Answer 1

STEP - BY - STEP EXPLANATION

What to find?

Equation of a line.

Given:

Perpendicular equation; y=-1/5 x - 3

Point(1,2)

Step 1

Find the slope of the perpendicular line.

Comparing the line with y=mx + c

`slope(m)=-(1)/(5)`

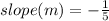
Step 2

Determine thee slope of the new equation.

Slope of perpendicular lines have the following characteristic;

`m_1m_2=-1`

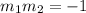
where m2 is the slope of the new equation.

`\begin{gathered} -(1)/(5)m_2=-1 \\ \\ m_2=-1*-(5)/(1) \\ \\ =5 \end{gathered}`

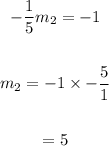
Step 3

Find the intercept(c) using the formula below:

`y=mx+c`

Substitute x=1 y=2 and m=5

`\begin{gathered} 2=5(1)+c \\ \\ c=2-5 \\ \\ =-3 \end{gathered}`

Step 4

Form the equation of the line by substituting m=5 and c=-3 into the general equation.

`y=5x+(-3)`

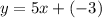
ANSWER

y= 5x + (-3)