Given
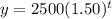
Find
Interpret the values of a and b , also annual percent increase
Step-by-step explanation
As the general form of growth exponential function is in the form of
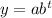
where a is the inital value
t is the time
b= 1+r = where r is the rate of growth
so , in given situation
a represents the number of referrals it received at the start of the model; and b represents the growth factor of the number of referrals
option 4 is the correct one.
now we have to find the annual percent increase
for this we have to find the final referrels after 1 years.
for this put t = 2in given equation
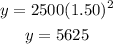
annual percent increase =
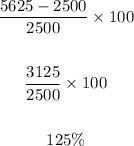
Final Answer
Therefore , the correct option is d .
the annual percent increase is 125%