Assume that the width of the rectangle = x
Since the length is twice the sum of the width and 3, then

Since the area of the rectangle is 216 square inches, then
Multiply the length and the width, then equate the product by 216

Divide all terms by 2 to simplify
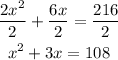
Subtract 108 from both sides
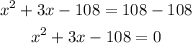
Now, let us factorize the trinomial into 2 factors
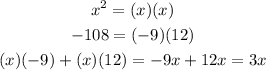
Then the factors are
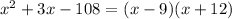
Equate them by 0
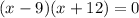
Equate each factor by 0, then find the values of x
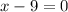
Add 9 to both sides
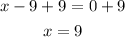
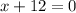
Subtract 12 from both sides
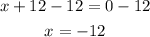
Since the width can not be a negative number (no negative length)
Then the width of the rectangle = 9
Let us find the length

Then the dimensions of the rectangle are 9 inches and 24 inches