Question

At an all-you-can-eat barbeque fundraiser, adults pay $6 for a dinner and children pay $4 for a dinner. 212 people attend and you raise $1,128. What is the total number of adults and the total number of children attending? A)140 adults and 72 children B)72 adults and 140 children C)142 adults and 70 children D)70 adults and 142 children

Answer 1

A)140 adults and 72 children

Step-by-step explanation

Step 1

Let x represents the number of childrend attending

Let y represents the number of adults attending

then

total cost for the children=4x

total cost for the adults=6y

if you raise 1128,

`4x+6y=1128\text{ Equation(1)}`

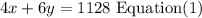
Now, 212 people attend,Hence

`x+y=\text{212 Equation(2)}`

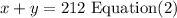
Step 2

solve for x and y

a)isolate x in equation (2), then replace in equation (1)

`\begin{gathered} x+y=212 \\ x=212-y \\ \text{now, replace} \\ 4x+6y=1128 \\ 4(212-y)+6y=1128 \\ 848-4y+6y=1128 \\ 2y+848=1128 \\ \text{subtract 848 in both sides} \\ 2y+848-848=1128-848 \\ 2y=280 \\ d\text{ivide boths ides by 2} \\ (2y)/(2)=(280)/(2) \\ y=140 \end{gathered}`

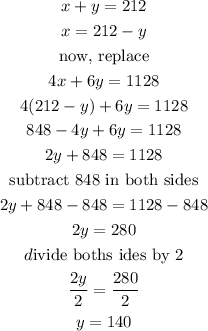
it means 140 adults are attending

b)replace y=140 in equatin (2) to find x

`\begin{gathered} x+y=212 \\ x+140=212 \\ \text{subtract 140 in both sides} \\ x+140-140=212-140 \\ x=72 \end{gathered}`

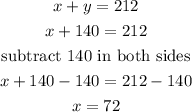
so, the number of children is 72 and 72 children