Question

Find an equation of the line. Write the equation using function notation.Through (4, - 7); perpendicular to 6y=x- 12The equation of the line is f(x)=..

Answer 1

To determine the equation of the line you need to determine its slope first.

You know that the line 6y=x-12 is perpendicular to the line you have to determine, two lines that are perpendicular, their slopes are opposite reciprocals. For example, let "m" represent the slope of one of the lines and "n" represent the slope of the perpendicular line, you can express their relationship as follows:

`m=-(1)/(n)`

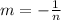
To determine the slope of the given line, you have to write it in slope-intercept form:

`y=mx+b`

Where

m represents the slope

b represents the y-intercept

Given the line:

`6y=x-12`

-Divide both sides by 6

`\begin{gathered} (6y)/(6)=-(x)/(6)=-(12)/(6) \\ y=-(1)/(6)x-2 \end{gathered}`

The slope of this line is the coefficient of the x-term, n=-1/6

Its opposite reciprocal is:

`\begin{gathered} m=-(1)/(n) \\ m=-(-(1)/((1)/(6))) \\ m=-(-1\cdot6) \\ m=-(-6) \\ m=6 \end{gathered}`

The slope of the line you have to determine is m=6

Now that you have the slope of the line, using the point-slope form, you can determine the equation of the line:

`y-y_1=m(x-x_1)`

Where

m represents the slope of the line

(x₁,y₁) represent the coordinates of one point of the line

Replace the formula with m=6 and (x₁,y₁)=(4,-7)

`\begin{gathered} y-(-7)=6(x-4) \\ y+7=6(x-4) \end{gathered}`

The next step is to write the equation in slope-intercept form:

-Distribute the multiplication on the parentheses term:

`\begin{gathered} y+7=6\cdot x-6\cdot4 \\ y+7=6x-24 \end{gathered}`

-Pass "+7" to the right side of the equation by applying the opposite operation "-7" to both sides of it:

`\begin{gathered} y+7-7=6x-24-7 \\ y=6x-31 \end{gathered}`

Finally, write the equation of the line using function notation:

`f(x)=6x-31`