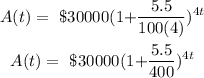The general formula for the amount in savings account compounded annually is given as;

A. The equation for the value of the investment as a function of t in the current account they have is;

B. The equation for the value of the investment in an account earning 5.875% interest compounded annually is;
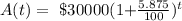
C. The equation for the value of the investment in an account earning 5.75% compounded semi-annually; that is twice in a year is;
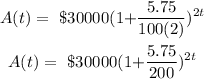
D. The solution for the value of the investment in an account earning 5.5% annual interest compounded quarterly; that is four times in a year;