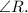Question

Question 7(Multiple Choice Worth 3 points)(05.04 LC)triangle PQR with side p across from angle P, side q across from angle Q, and side r across from angle RIf ∠R measures 18°, q equals 9.5, and p equals 6.0, then which length can be found using the Law of Cosines? p q RQ PQ

Answer 1

Using the Law of Cosines, the length that can be found is
`\( PQ \).`

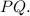
Step-by-step explanation:

The Law of Cosines is expressed as:

`\[ c^2 = a^2 + b^2 - 2ab \cos(C) \]`

where c is the side length across from angle C , and a and b are the other two side lengths of the triangle.

In the given triangle PQR , if angle R measures 18°, and q and p are known, we can use the Law of Cosines to find the length of side PQ , which is opposite to angle R .

Let
`\( PQ = c \), \( PR = a \), and \( QR = b \).`

`\[ PQ^2 = PR^2 + QR^2 - 2 \cdot PR \cdot QR \cdot \cos(\angle R) \]`

Substituting the given values:

`\[ PQ^2 = p^2 + q^2 - 2 \cdot p \cdot q \cdot \cos(18°) \]`

Now, calculate the numerical value of PQ using the provided values of p , q , and
`\( \angle R \).`

Answer 2

PQ

Explanation

It must be PQ because we have the measure of the other two sides and the angle opposite it.