We have the function:
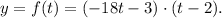
a. Zeros of the function
By definition, the zeros are the values of t such that f(t) = 0. In this case, we have:

Rewriting the function, we have:
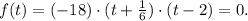
So the zeros of the function are:
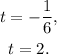
b. Meaning of the zeros
The function y = f(t) represents the height of the ball at time t.
• So the zeros are the times at which the function reaches a height equal to zero.
,
• We see that one zero is positive and the other negative. Only the positive zero (t = 2) is meaningful because the negative (t = -1/6) represents a negative value of time!
c. Initial height
The ball is thrown at time t = 0. The height of the ball at time t = 0 is:
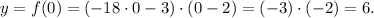
So the ball is thrown from a height of 6 feet.
Answer
• a., The zeros are t = -1/6 and t = 2.
,
• b., The zeros are the values of time at which the height of the ball is zero. Only a positive value of time makes sense, so only the zero t = 2 is meaningful.
,
• c., The ball is thrown from a height of 6 feet.