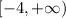a. Graph -f(x):
By the transformations rules for functions, the graph of -f(x) is equal to a reflection over the x-axis, and a change of the y-coordinates:
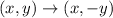
Then, given the function:
![f(x)=\sqrt[]{x}](https://img.qammunity.org/2023/formulas/mathematics/college/eoshqhavec9ovuzgludbh20udpt0k7hgf6.png)
The graph of -f(x) is:is
The domain of the function is the set of all possible x-values, then it is:
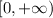
The range is the set of all possible values of the function, then it is:
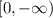
b. Graph f(x+2)-4:
The transformation f(x+2) is an horizontal translation left 2 units.
And the transformation f(x+2)-4 is a vertical translation down 4 units.
Then, the coordinates of this graph in comparison to the given graph are:

Then for the point (1,1) the new coordinates are (1-2,1-4)=(-1,-3).
For (4,2): the new coordinates (4-2,2-4)=(2,-2)
For (9,3): the new coordinates (9-2,3-4)=(7,-1)
The graph is:
The domain of this function is:

And the range is: