SOLUTION
Given the image in the question tab, the following are the solution steps to the answer
Step 1: Write out the function
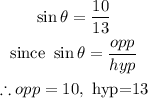
Step 2: Solve for the adjacent using the pythagoras theorem
![\begin{gathered} \text{hyp}^2=opp^2+adj^2 \\ 13^2=10^2+adj^2 \\ \text{adj}^2=13^2-10^2 \\ \text{adj}=\sqrt[]{169-100} \\ \text{adj}=\sqrt[]{69} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/z27mdbn1hy29xbstm1kjkf2p11zbx2nkxk.png)
Step 3: Calculate the value of cos2Ф
![\begin{gathered} cos2\theta=\cos ^2\theta-\sin ^2\theta \\ \cos 2\theta=(\frac{\text{adj}}{\text{hyp}})^2-((opp)/(hyp))^2 \\ \cos 2\theta=(\frac{\sqrt[]{69}}{13})^2-((10)/(13))^2 \\ \cos 2\theta=(69)/(169)-(100)/(169) \\ \cos 2\theta=-(31)/(169) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/v4utydpkdwzmd53se7ikzxiy17ngths32d.png)
Hence, the value of cos2Ф is -31/169.