Question

A convention center is in the shape of the rectangular pyramid with a height of 444 yd. Its base measures 348 yd by 418 yd. Find the volume of the convention center. If necessary, round your answer to the nearest tenth.

Answer 1

Given:

Length of the base = 418 yd

Width of the base = 348 yd

Height of the pyramid = 444 yd

Find: Volume of the rectangular pyramid

Solution:

The formula to get the volume of the rectangular pyramid is:

`V=(1)/(3)\text{Area of the base}* height`

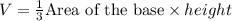
Since the base is rectangular, we can replace the "area of the base" into "length x width" since that is the formula for the area of a rectangle.

`V=(1)/(3)l* w* h`

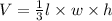
Let's plug in the given data to the formula above.

`V=(1)/(3)418yd*348yd*444yd`

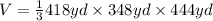
Then, solve for V or volume.

`\begin{gathered} V=(1)/(3)*64,586,016yd^3 \\ V=21,528,672yd^3 \end{gathered}`

Answer: The volume of the convention is 21, 528, 672 yd³.