Question

I wills send you a picture

Answer 1

Draw the tank

we can use the formula of the volume of a cylinder

`V=\pi* r^2* h`

we can repalce the value of the volume (320pi) and the height

`\begin{gathered} 320\pi=\pi* r^2*20 \\ 320\pi=20r^2\pi \end{gathered}`

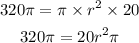
now solve for r^2 dividing 20pi on both sides

`\begin{gathered} (320\pi)/(20\pi)=r^2 \\ \\ r^2=16 \\ \end{gathered}`

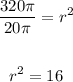
and solve for r using roots

`\begin{gathered} r=\sqrt[]{16} \\ \\ r=4 \end{gathered}`

the value of the radious is 4ft and the diameter double, then

`\begin{gathered} d=2*4 \\ d=8 \end{gathered}`

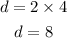
diameter of the cylinder is 8 ft then rigth option is C