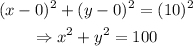ANSWER
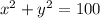
Step-by-step explanation
The general equation of a circle is:

where (h, k) = the center of the circle
r = radius of the circle (i.e. distance from any point on its circumference to the center of the circle)
The center of the circle is the origin, that is:
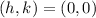
To find the radius, apply the formula for distance between two points:
![r=\sqrt[]{(x_1-h)^2+(y_1-k)^2_{}}](https://img.qammunity.org/2023/formulas/mathematics/college/17gvphrzlc8aelwve35aipyv595n13mqlw.png)
where (x1, y1) is the point the circle passes through
Hence, the radius is:
![\begin{gathered} r=\sqrt[]{(0-0)^2+(-10-0)^2}=\sqrt[]{0+(-10)^2} \\ r=\sqrt[]{100} \\ r=10 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/j9jz9x0uj065qjk8q4gla0hrn2e1bs64iq.png)
Hence, the equation of the circle is: