The point of the problem is to remember the cosine relation. It says, in this case, that

Converting the last equation by the inverse function, we get
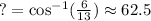
For the first decimal place (5) equals 5, and by the rounding rule to the nearest degree, we get 63. The answer is A.