Question

1. A trapezoid is pictured on the right. Its bottom base a is not changing while its top base b is increasing at the rate of 3 inches per second while its height h is decreasing at the rate of 0.5 inches per second. How fast is the area of the trapezoid changing, measured in square inches per second, at this moment?

A) -2.5
B) -.75
C) 1
D) 2
(Please explain)

Answer 1

the answer is A...

Explanation:

Calculate Area (A):

The area formula for a square is denoted below:

A = s2

Taking the square root of both sides, we get:

s = √A

s = √3

s = 1.7320508075689

Answer 2

The area of the trapezoid is changing at the rate of
`\( 1 \)`square inch per second at this moment. The correct answer is C) 1.

The area
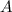`\( A \)` of a trapezoid can be found using the formula:

`\[ A = (1)/(2) * (a + b) * h \]`

Given that the top base
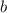`\( b \)` is increasing at the rate of
`\( (db)/(dt) = 3 \)` inches per second and the height
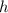`\( h \)` is decreasing at the rate of
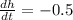`\( (dh)/(dt) = -0.5 \)` inches per second, we want to find the rate of change of the area
`\( (dA)/(dt) \)` at the moment when
`\( b = 4 \)` inches,
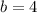`\( h = 3 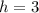\)` inches, and
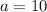`\( a = 10 \)` inches.

Differentiate the area formula with respect to time
`\( t \)`:

`\[ (dA)/(dt) = (1)/(2) * \left( (d)/(dt)(a + b) * h + (a + b) * (dh)/(dt) \right) \]`

Since
`\( a \)` is not changing,
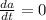`\( (da)/(dt) = 0 \)`, and the formula simplifies to:

`\[ (dA)/(dt) = (1)/(2) * ( (db)/(dt) * h + (a + b) * (dh)/(dt)) \]`

Now we can plug in the given rates and values:

`\[ (dA)/(dt) = (1)/(2) * (3 * 3 + (10 + 4) * -0.5) \]`

`\[ (dA)/(dt) = (1)/(2) * (9 + 14 * -0.5) \]`

`\[ (dA)/(dt) = (1)/(2) * (9 - 7) \]`

`\[ (dA)/(dt) = (1)/(2) * 2 \]`

`\[ (dA)/(dt) = 1 \]`

Therefore, the area of the trapezoid is changing at the rate of
`\( 1 \)`square inch per second at this moment. The correct answer is C) 1.