Vertical asymptotes are when the denominator is 0 but the numerator isn't 0.
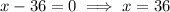
Since this value of x does not make the numerator equal to 0, the vertical asymptote is
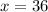 .
.
Horizontal asymptotes are the limits as
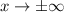 .
.
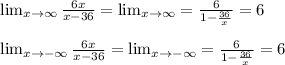
So, the horizontal asymptote is
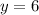 .
.
End behavior:
- As

- As
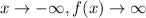 .
.