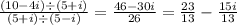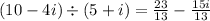 , which can be further simplified if needed.
, which can be further simplified if needed.
To evaluate the expression
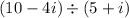 , we'll multiply both the numerator and denominator by the conjugate of the denominator to rationalize the denominator.
, we'll multiply both the numerator and denominator by the conjugate of the denominator to rationalize the denominator.
The conjugate of
 .
.
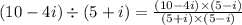
Let's perform the multiplication:
Numerator:
![\[ (10 - 4i) * (5 - i) = 50 - 10i - 20i + 4i^2 \]](https://img.qammunity.org/2023/formulas/mathematics/college/9laxt3h5abjv4rqgxwrp853oix9zmdsw5v.png)
![\[ = 50 - 30i - 4 \] (Remember that \(i^2 = -1\))](https://img.qammunity.org/2023/formulas/mathematics/college/2nlyl9z6k6v70zbj1smqw4enlzsgbd7bsv.png)
![\[ = 46 - 30i \]](https://img.qammunity.org/2023/formulas/mathematics/college/k4q6t52il2whz1lsqae5x2zn3adsjvc4ez.png)
Denominator:
![\[ (5 + i) * (5 - i) = 25 - 5i + 5i - i^2 \]](https://img.qammunity.org/2023/formulas/mathematics/college/h63rlnow9dmbee79j5ndtko1qa126s6fr7.png)
![\[ = 25 - i^2 \]](https://img.qammunity.org/2023/formulas/mathematics/college/5o1oi2p5qxta20o3d2nuqm206w6adjtqpf.png)
![\[ = 25 + 1 \]](https://img.qammunity.org/2023/formulas/mathematics/college/34fkkedwyzsafl5c4w0tn47xys3m72knqr.png)
![\[ = 26 \]](https://img.qammunity.org/2023/formulas/mathematics/college/qbw1esdveltx2nukc3uh19ognasysyxzpw.png)
Now, simplify the expression: