Question

What is the value of f(x)=2(0.75)^x when x=3

Answer 1

Answer: f(3) = 0.84375

Explanation:

f(3) is asking for the value of the function when x = 3, not multiplying f by 3. (f(x) is basically the output, y)

So we just plug in 3 for x and simplify (use PEMDAS)

f(3) = 2(0.75)^3

f(3) = 2(0.421875)

f(3) = 0.84375

Answer 2

`(27)/(32)`

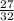
Explanation:

It may be a bit easier to represent 0.75 as a fraction, since we can use the following property of exponents:
`((a)/(b))^x = (a^x)/(b^x)`

We can represent 0.75 as:
`(3)/(4)`

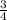
And substituting this into the function we get:
`f(x)=2((3)/(4))^x`

calculating the value when x=3, we get:
`f(3)=2((3)/(4))^3`

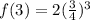
using the property previously stated we get:
`f(3)=2((3^3)/(4^3))`

Raising each value to the exponent we get:
`f(3)=2((27)/(64))`

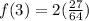
When we multiply by two, we can simplify by splitting up 64, into two factors: 2 and 32, to get:
`(2*27)/(32*2)`

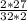
From here it's easy to see that we can cancel out the values 2, to get:
`(27)/(32)`

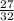
Which is our answer!