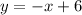Slope Intercept Form From a Graph
Slope intercept form:

We can identify two points on the given graph and solve for the slope and the y-intercept.
Solving the Question
Two clear points on the graph are (6,0) and (0,6).
First, we can solve for the slope by using the slope equation:
 where two points are
where two points are
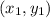 and
and
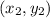
⇒ Plug in the points (6,0) and (0,6):

Therefore. the slope of the line is -1. Plug this into
 :
:
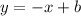
Now, we can find the y-intercept. It is the value of y when the line crosses the y-axis.
In this case, we can see on the graph that this is 6.
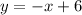
Answer