Answer:
y = -19
Explanation:
Pre-Solving
We are given two points: (1, y) and (8,2).
We want to find the value of y if the slope of the line is 3.
Solving
The slope (m) can be calculated from two points using the formula
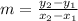 where
where
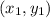 and
and
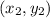 are points
are points
We can label the values of the points to avoid any confusion and mistakes.
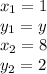
Substitute these values into the formula.
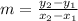

Remember that the slope of the line is 3, so we can substitute m as 3.
Replace m as 3.
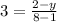
Subtract.
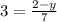
Multiply both sides by 7.

21 = 2-y
Subtract 2 from both sides.
19 = -y
Divide both sides by -1.
-19 = y
y = - 19.