Question

Help please I did them and got all wrong LOL..............

Answer 1

1. Choice (2) 13

2. Choice (3) 8.1

3. Choice (3) 95 to 105 ft

4. Choice (3) 96 in

Explanation:

All the problems use the Pythagorean theorem
The Pythagorean Theorem states that the sum of the squared sides of a right triangle equals the length of the hypotenuse squared.

`c^(2) = a^(2) + b^(2)`

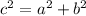
or

`c = \sqrt{a^(2) + b^(2)}`

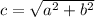
where c is the hypotenuse and a, b the shorter sides.

This means that given any two of the three sides of a right triangle we can compute the length of the third side

For example if we were given the hypotenuse c and side b, we can solve for side a by:

`a = \sqrt{c^(2) - b^(2)}`

If we were given side a and asked to solve for side b then
b = \sqrt{c^{2} - a^{2}}

Frankly it does not matter which you choose as side a and side b.

Question 1
The distance from the foot of the ladder to the wall can be taken to be side a and is equal to 8ft

So b = 8ft

The length of the ladder is the hypotenuse c = 15 feet

`a = \sqrt{c^(2) - b^(2)} \\\\a = \sqrt{15^(2) - 8^(2)}\\\\a = √(225 - 64)\\\\a = √(161)\\\\a = 12.68857754045 \\\\`

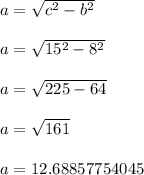
Rounded to nearest foot, that would be 13 feet So choice (2)

Question 2

The points J and K have the following coordinates as indicated on the graph.

J(-3, 2)

K (1, -5)

The distance between two points is the length of the path connecting them. The shortest path distance is a straight line. In a 2 dimensional plane, the distance between points (X1, Y1) and (X2, Y2) is given by the Pythagorean theorem:

`d = \sqrt {(x_(2) - x_(1))^2 + (y_(2) - y_(1))^2}`

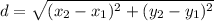
For:

(X1, Y1) = (-3, 2)

(X2, Y2) = (1, -5)

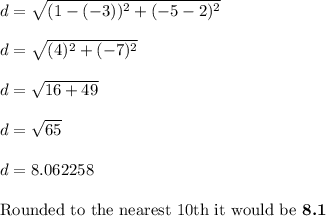`d = \sqrt {(1 - (-3))^2 + (-5 - 2)^2}\\\\d = \sqrt {(4)^2 + (-7)^2}\\\\d = \sqrt {{16} + {49}}\\\\d = \sqrt {65}\\\\d = 8.062258\\\\\text{Rounded to the nearest 10th it would be \boldsymbol{8.1}}\\\\` So choice (3)

Question 3

This again involves a right triangle as shown in the figure
The sides are a = AC = 60 and b = BC = 80 and we are asked to find the length of AC which is the hypotenuse of ΔABC

Use the Pythagorean Theorem directly

`c = \sqrt{a^(2) + b^(2)}}\\\\a = \sqrt{60^(2) + 80^(2)}\\\\c = √(3600 + 6400)}\\\\c = √(10000)}\\\\c = 100}\\\\`

Answer 100 feet so choice (3): from 95 to 105 ft

Question 4

The brace is one of the shorter sides, with the platform top as the hypotenuse.

Let's use a for the brace, b for the 40 in side and c for the hypotenuse = 104 in

So we have to compute for b using the formula:

`b = \sqrt{c^(2) - a^(2)}`

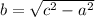
Using the given values, this would be:

`b = \sqrt{104^(2) - 40^(2)}\\\\b = √(10816 - 1600)\\\\b = √(9216)\\\\b = 96\\\\`

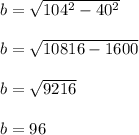
which would be choice (3)