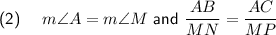Question

Which of the following would be enough information to know that ABC~MNP?

Answer 1

`\textsf{(2) \quad $m \angle A=m \angle M$ and $(AB)/(MN)=(AC)/(MP)$}`

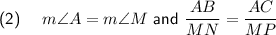
Explanation:

Two triangles are said to be similar if their corresponding angles are the same size and the corresponding sides are in the same ratio.

Therefore, if triangle ABC is similar to triangle MNP then their corresponding angles are congruent:

• m∠A = m∠M
• m∠B = m∠N
• m∠C = m∠P

Similarly, if triangle ABC is similar to triangle MNP then their corresponding sides are in the same ratio:

`\implies (AB)/(MN)=(BC)/(NP)=(AC)/(MP)`

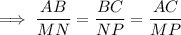
SAS Triangle Similarity

If two sides of one triangle are in the same ratio to the corresponding sides of another triangle, and their included angles are congruent, then the triangles are similar.

∠A is the included angle of sides AB and AC.

∠M is the included angle of sides MN and MP.

Therefore, the only statement that shows that two corresponding sides are in the same ratio and their included angles are congruent is:

`\textsf{(2) \quad $m \angle A=m \angle M$ and $(AB)/(MN)=(AC)/(MP)$}`