8 hours
Step-by-step explanation
Step 1
Let
distance = 239000 miles
speed = 500 miles per minute
time( hours) = t
Step 2
to find the time, use
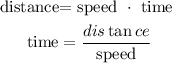
replace
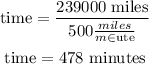
Hence, time = 478
Step 2
convert the minutes into hours
if 1 hour = 60 minutes
then, x=hurs for 478 minutes

Now, solve for x

Step 3
Round to the nearest hour
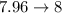
8 hours