Answer:
32 square units
Explanation:
A plot of the points shows that PQRS is a rectangle.
The length of this is the distance of the segment PQ = distance of segment RS = 6.4 units
The width QR = width PS = 5 units
The area is 5 x 6.4 = 32 square units
Lengths are calculated as follows
Finding length of PQ using distance formula:
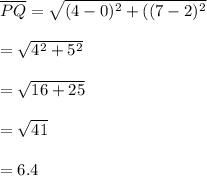
Finding length of QR using distance formula:
