Question

joe is climbing the ladder and stops when his feet are vertically 3.2 feet above the ground and horizontally 2.4 feet from the base of the ladder. Which equation represents this new situation and can be used to find how far Joe climbed up the ladder? Select the correct answer. 3.2 + 2.4 = c a2 + 2.42 = 3.22 3.22 + b2 = 2.42 3.22 + 2.42 = c2

Answer 1

Answer: (3.2) + (2.4)2 = (c)2

Step-by-step explanation:

An image is attached with it.

Lets say that AB is the wall and AC measuring (c) feet is the ladder length.

Now as Joe is climbing on it.

It moves horizontally 2.4 ft taking it as x - axis direction from the base C then it also climb upward 3.2 ft considering it as y-axis.

We see that the situation where we have drawn the ladder and the support (wall) it forms a right angled triangle.

So for this, the ladder is equivalent to the hypotenuse of the triangle. And from Pythagoras formula for a right angled triangle we know that:

`(Hypotenuse)^2 = (Base)^2 + (Perpendicular)^2`

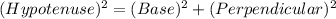
Here the base = 2.4 ft and perpendicular = 3.2 ft.

So the hypotenuse/ladder which is - (c)2 = (2.4)2 + (3.2)

The situation which can be used to find how far Joe has climbed up the ladder = (c)2 = (2.4)2 + (3.2)2